Question

What is the acceleration of a proton moving with a speed of 7.7 m/s at right angles to a magnetic field of 1.9 T

Answer 1

The acceleration of the proton is 1.403 x 10⁹ m/s²

Step-by-step explanation:

Given;

speed of proton, v = 7.7 m/s

magnitude of magnetic field, B = 1.9 T

Magnetic force of moving proton is given by;

F = qvBsinθ

Centripetal force on the moving proton is given by;

`F = m((v^2)/(r))\\\\F = m(a_c) \\\\a_c \ is \ the \ centripetal \ acceleration`

`qvBsin\theta = ma_c\\\\ac = (qvBsin(90))/(m)`

where;

q is charge of the proton = 1.602 x 10⁻¹⁹ C

m is mass of proton = 1.67 x 10⁻²⁷ kg

`ac = ((1.602*10^(-19))(7.7)(1.9)sin(90))/(1.67*10^(-27))\\\\a_c = 1.403*10^(9) \ m/s^2`

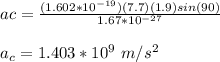
Therefore, the acceleration of the proton is 1.403 x 10⁹ m/s²