Question

What are the distances from the point (x,y) to the focus of the parabola and the directrix? Select two answers. A.distance to the directrix: |y+6| B.distance to the focus: (x+4)2+(y−2)2√ C.distance to the directrix: |y−6| D.distance to the focus: (x−2)2+(y+4)2√ E.distance to the directrix: |x+6| F.distance to the focus: (x−2)2+(y+5)2√

Answer 1

Option (A) and Option (D)

Explanation:

Point on the parabola is (x, y).

Focus given as (2, -4) and directrix of the parabola is y = -6

Therefore, distance of the point from the directrix will be,

d = |(y + 6)|

Similarly, distance of the point (x, y) from the focus will be,

d =
`√((x_2-x_1)^2+(y_2-y_1)^2)`

=
`√((x-2)^2+(y+4)^2)`

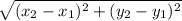
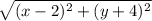
Therefore, Option (A) and Option (D) will be the correct options.