Answer:
The probability that exactly five of the seven have straight stitching is very low only 13.47%, this means that the company should stop the production line.
Explanation:
We are given that Seven baseballs are randomly selected from the production line to see if their stitching is straight. Over time, the company has found that 89.4% of all their baseballs have straight stitching.
Let X = Number of baseballs having straight stitching
The above situation can be represented through the binomial distribution;
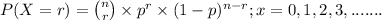
where, n = number of samples (trials) taken = 7 baseballs
r = number of success = exactly 5
p = probbaility of success which in our question is the probability
that baseballs have straight stitching, i.e.; p = 89.4%
So, X ~ Binom(n = 7, p = 0.894)
Now, the probability that exactly five of the seven have straight stitching is given by = P(X = 5)
P(X = 5) =
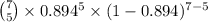
=
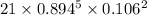
= 0.1347 or 13.47%
Since the probability that exactly five of the seven have straight stitching is very low only 13.47%, this means that company should stop the production line.