Question

Two long parallel wires are separated by 6.0 mm. The current in one of the wires is twice the other current. If the magnitude of the force on a 3.0-m length of one of the wires is equal to 8.0 μN, what is the greater of the two currents?

Answer 1

The greater of the two currents is 0.692 A

Step-by-step explanation:

Given;

distance between the two parallel wires; r = 6 mm = 6 x 10⁻³ m

let the current in the first wire = I₁

then, the current in the second wire = 2I₁

length of the wires, L = 3.0 m

magnitude of force on the wires, F = 8 μN = 8 x 10⁻⁶ N

The magnitude of force on the two parallel wires is given by;

`F = (\mu_o I_1(2I_1))/(2\pi r)\\\\F = (\mu_o 2I_1^2)/(2\pi r)\\\\I_1^2 = (F*2\pi r)/(2\mu_o) \\\\I_1^2 = (8*10^(-6)*2\pi (6*10^(-3)))/(2(4\pi*10^(-7)))\\\\I_1^2 = 0.12\\\\I_1 = √(0.12)\\\\ I_1 =0.346 \ A`

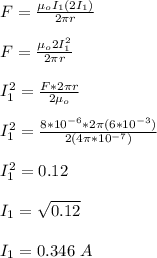
the current in the second wire = 2I₁ = 2 x 0.346 A = 0.692 A

Therefore, the greater of the two currents is 0.692 A