Answer:
The answer is below
Explanation:
a) For a normal model the sample size has to be equal or greater than 30 so that it can be a normal distribution.
b) Given that:
μ = 11.2 minutes, σ = 4.8 minutes, n = 45
The z score determines how many standard deviations the raw score is above or below the mean. It is given by:
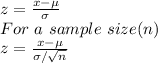
For x < 10 minutes
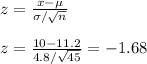
Therefore from the normal distribution table, P(x < 10) = P(z < -1.68) = 0.0465