Answer and Explanation:
a. The computation of the economic order quantity is shown below:

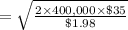
= 3,761 units
b. The number of orders would be equal to
= Annual demand ÷ economic order quantity
= 400,000 ÷ 3,761 units
= 106.35 orders
c. The computation of the total cost is shown below:
= Purchase cost + ordering cost + carrying cost
where,
Purchase cost = Annual consumption × Cost per unit
= 400,000 × $9
= $2,800,000
Ordering cost = (Annual demand ÷ EOQ) × Cost to place one order
= (400,000 ÷ 3,761) × $35
= $3,723
Carrying cost = (EOQ ÷ 2) × carrying cost percentage × Cost per unit
= (3,761 ÷ 2) × 22% × $9
= $3,723
Now put these values to the above formula
So, the value would equal to
= $2,800,000 + $3,723 + $3,723
= $2,807,446