Question

The ratio of the areas of two similar

parallelograms is 4:9. Find the height of the
bigger one if the smaller is of height 4cm.
​

Answer 1

Answer: 6 cm

Explanation:

Given: The ratio of the areas of two similar parallelograms is 4:9.

To find : The height of the bigger one if the smaller is of height 4 cm.

Let h be the height of the bigger one.

Since the areas of similar figures are proportional to the square of their corresponding sides.

Then,
`(4^2)/(h^2)=(4)/(9)`

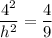
`(16)/(h^2)=(4)/(9)\\\\\Rightarrow\ h^2=(9)/(4)*16\\\\\Rightarrow\ h^2=36\\\\\Rightarow\ h= 6\ cm\ \ \ \ \text{[ height cannot be negative.]}`

Hence, the height of bigger parallelogram = 6 cm