Question

Determine whether the sum of two values is a rational number or an irrational number.

Answer 1

1). Rational

2). Irrational

3). Rational

4). Rational

5). Irrational

Explanation:

To solve this question we should follow the rule,

"Addition of rational and an irrational number is an irrational number."

1).
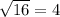`√(16)=4` (rational number)

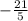`-(21)/(5)` (negative rational number)

`√(16)-(21)/(5)=4-(21)/(5)`

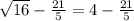
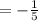`=-(1)/(5)` [rational number]

2). π → (Irrational number)

24 → (rational number)

π + 24 → (Irrational number)

Since addition of a rational and an irrational number is an irrational number.

3).
`√(4)` = 2 (rational number)

5 (rational number)

2 + 5 = 7 (rational number)

4).
`√(36)=6` (rational number)

`\sqrt[3]{27}=3` (rational number)

`√(36)+\sqrt[3]{27}=9` (rational number)

5).
`(3)/(4)` (rational number)

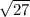`√(27)` → (Irrational number)

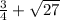`(3)/(4)+√(27)` → (Irrational number)