Question

You have a 100-kg object sitting on a frictionless tabletop. The object is connected to a spring with k = 1000 N/m and a natural length of 1 m with the other end of the spring connected to the wall. You pull the object 50 cm from the equilibrium position of the spring and hold it in place, and then release it.

Required:
a. How hard were you pulling on the object to hold it in place?
b. How much work did you do to move the object to that spot?
c. How close to the wall will the object get?
d. What is the fastest that the object moves and where is that location?

Answer 1

a) 500 N

b) 250 J

c) 0.87 m

d) 1.58 m/s, at 0.6 m from the wall

Step-by-step explanation:

The mass of the object m = 100 kg

the spring constant k = 1000 N/m

length of the the spring = 1 m

extension of the string = 50 cm = 0.5 m

a) Force used to pull the mass is gotten from Hooke's law equation

F = -kx

where F is the force used to pull = ?

k is the spring constant = 1000 N/m

x is the extension = 0.5 m

substituting, we have

F = 1000 x 0.5 = 500 N this force is used to pull the mass

b) The work done in moving the mass = Fx

==> 500 x 0.5 = 250 J

c) The energy stored up in the spring U =
`(1)/(2)kx^(2)`

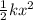
U =
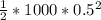`(1)/(2)*1000*0.5^(2)` = 125 J

energy available for the mass from its equilibrium position = 250 - 125 = 125 J

this energy is equivalent to the work done by the spring on the mass by moving it closer to the wall

Work W = (weigh of the mass) x distance moved

weight = mg

where m is the mass = 100 kg

g is acceleration due to gravity = 9.81 m/s^2

substituting, we have

W = mgd

where d is the distance the mass moves closer to the wall

W = 100 x 9.81 x d

but W = 125 J

125 = 981d

d = 125/981 = 0.13 m

closeness to the wall = L - d

where L is the natural length of the spring = 1 m

closeness to the wall = 1 - 0.13 = 0.87 m

d) The maximum kinetic energy of the object will be halfway between the extended length and the final resting place.

extended length = 1 + 0.5 m = 1.5 m

distance from resting place = 1.5 - 0.87 = 0.63 m from the wall

At this point, all the mechanical energy on the mass and spring system is converted to kinetic energy of motion.

KE =
`(1)/(2)mv^(2)`

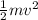
substituting,

125 =
`(1)/(2)*100*v^(2)` =
`50v^(2)`

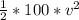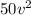
`v^(2)` = 125/50 = 2.5

v =
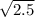`√(2.5)` = 1.58 m/s