Answer:
Step-by-step explanation:
a )
focal length of convex spherical mirror
f = 36/2 cm = 18 cm
object distance u = - 25 cm
mirror formula
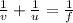
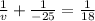
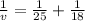
v = 6.28 cm .
It is positive hence the image will be erect / upright and formed on the back of the mirror.
For object distance of 47 cm
u = - 47 cm
Putting the values in the mirror formula
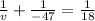
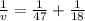
v = 13 cm
It is positive hence the image will be erect / upright and formed on the back of the mirror.