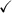Question

Please help me!!!!!!!!!!!!!​

Answer 1

Answer: see proof below

Explanation:

Use the following Half-Angle Identities: tan (A/2) = (sinA)/(1 + cosA)

cot (A/2) = (sinA)/(1 - cosA)

Use the Pythagorean Identity: cos²A + sin²B = 1

Use Unit Circle to evaluate: cos 45° = sin 45° =
`(\sqrt2)/(2)`

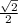
Proof LHS → RHS

Given:
`cot\ (22(1)/(2))^o-tan\ (22(1)/(2))^o`

Rewrite Fraction:
`cot\ ((45)/(2))^o-tan\ ((45)/(2))^o`

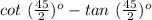
Half-Angle Identity:
`(sin(45)^o)/(1-cos(45)^o)-(sin(45)^o)/(1+cos(45)^o)`

Substitute:
`((\sqrt2)/(2))/(1-(\sqrt2)/(2))-((\sqrt2)/(2))/(1+(\sqrt2)/(2))`

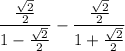
Simplify:
`((\sqrt2)/(2))/((2-\sqrt2)/(2))-((\sqrt2)/(2))/((2+\sqrt2)/(2))`

`=(\sqrt2)/(2-\sqrt2)-(\sqrt2)/(2+\sqrt2)`

`=(\sqrt2)/(2-\sqrt2)\bigg((2+\sqrt2)/(2+\sqrt2)\bigg)-(\sqrt2)/(2+\sqrt2)\bigg((2-\sqrt2)/(2-\sqrt2)\bigg)`

`=(2\sqrt2+2)/(4-2)-(2\sqrt2-2)/(4-2)`

`=(4)/(2)`

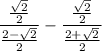
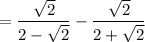
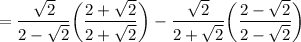
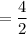
= 2

LHS = RHS: 2 = 2
`\checkmark`