Question

Each product is in the form ax^2+ by+c. Which of the following explains the relationship between b and c?

Answer 1

c is the square of half of b

Explanation:

A quadratic equation can be written in the following form:

`ax^2+bx+c`.

Based off of the information provided, we can test to see if which answer choice is correct.

OPTION A IS INCORRECT:

Although, for
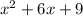`x^2+6x+9`, c is 1.5 times b, we do not see that relationship reflected in other equations, such as
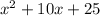`x^2+10x+25` and
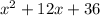`x^2+12x+36`. There must be a different relationship.

OPTION B IS INCORRECT:

Although c is double b for x^2+8x+16, this rule is not applicable for the rest of the equations. C is double b for the is 1.5 times that of b for x^2+6x+9, and for and for x^2+10x+25, c is 2.5 times b, and for x^2+12x+36, c is 3 times of b.

OPTION C IS CORRECT:

We can test this relationship based off of the information given.

for x^2+6x+9, c equals (6/2)^2 which is 9

for x^2+8x+16, c equals (8/2)^2 which is 16

for x^2+10x+25 c equals (10/2)^2 which is 25

and finally, for x^2+12x+36 c equals (12/2)^2 which is 36.

OPTION D IS INCORRECT:

C is not the square of B.

for x^2+6x+9, b^2 which is 36 (c here is 9)

for x^2+8x+16, b^2 is 64 (c here is 16)

for x^2+10x+25 b^2 is 100 (c here is 25)

and finally, for x^2+12x+36 b^2 is 144 (c here is 36).