Answer:
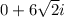
or just
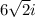
Explanation:
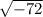 does not have a real part, it is a pure imaginary number.
does not have a real part, it is a pure imaginary number.
Let's simplify.
First step:
 is the imaginary unit.
is the imaginary unit.
So we have that we can write
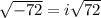 .
.
Second step:
Let's simplify the factor
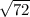 by looking for perfect squares of
by looking for perfect squares of
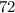 .
.

So
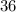 is a perfect square because it can be written as
is a perfect square because it can be written as
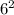 .
.
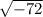
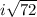
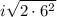
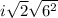
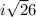

We could write this as
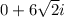 .
.