Question

EXAMPLE 11 Show that the points (1,-1),(5,2) and (9,5) are collinear​

Answer 1

Explanation:

Hey, there!!!

Your question is about showing the points A(1,-1), B(5,2) and C(9,5) as a collinear point.

We generally slope to find weather the points are collinear or not.

So, let's find slope for AB.

`slope(m) = (y2 - y1)/(x2 - x1)`

`slope = (2 + 1)/(5 - 1)`

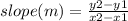
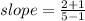
Therefore, slope of AB = 3/4.

Now, slope of BC.

`slope(m) = (y2 - y1)/(x2 - x1)`

`slope \: (m) = (5 - 2)/(9 - 5)`

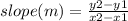
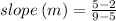
Therefore, the slope is 3/4.

now, lastly slope of AC.

`slope(m) = (y2 - y1)/(x2 - x1)`

`slope(m) = (5 + 1)/(9 - 1)`

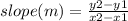
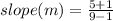
Therefore, the slope of AC is 3/4.

As all point have same slope. They are collinear point.

Hope it helps...

Answer 2

see explanation

Explanation:

They are collinear if the have the same slope

(2 - -1)/(5 - 1) = 3/4

(5 - 2)/(9 - 5) = 3/4

(5 - -1)/(9 - 1) = 6/8 = 3/4