Answer: 2.308 .
Explanation:
Let X denotes the number of earthquakes in SanFernando valley region of Los Angeles in 1994.
Given:

Probability is 0.87 that there will be at least 15 earthquakes .
i.e.
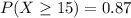
![\Rightarrow\ P((X-\mu)/(\sigma)\geq(15-17.6)/(\sigma))=0.87\\\\ \Rightarrow\ P(Z\geq(-2.6)/(\sigma))=0.87\ \ \ [Z=(X-\mu)/(\sigma)]](https://img.qammunity.org/2021/formulas/mathematics/college/hezwq35do32e0p7y4yju0tlvdh7k838ird.png)
Z-value corresponding to p-value 0.87 is -1.1263 .
So,
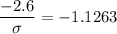
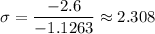
Hence, the required standard deviation = 2.308 .