Answer:
The volume of the triangular prism is not equal to the volume of the cylinder
Explanation:
Given that:
The cross sectional area of triangular prism and a night cylinder are congruent. The term 'congruent' means when two objects or shapes are placed above each other , they should both overlap exactly.
It was given that:
The triangular prism has a height of 5 units
and the right cylinder height of 3 units
The conclusion that can be drawn from this is that:
The volume of the triangular prism is not equal to the volume of the cylinder.
This is because, they do not occur in the same family. A triangular prism is a three - dimensional shape that has identical, parallel ends and parallel faces.
A triangular prism has two triangles at it ends and three rectangular faces.
The volume of the triangular prism is calculate by using the formula:
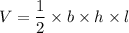
On the other hand, a cylinder is a shape with two circular ends and a curved surface.The two circular ends are congruent and both circular end posses the same cross section from one end to the other in the cylinder.
The volume of a cylinder is calculated by using the formula:
V = πr²h
Thus, we conclude that irrespective of the nature of their congruency, since the heights are different which will definitely affects their volume, the the volume of the triangular prism is not equal to the volume of the cylinder.