Question

Suppose you have a pocketful of change. You have some pennies (p) and some quarters (q). One expression could be used to describe the total number of coins in your pocket: p + q. A second expression could be used to describe the amount of money (in dollars) in your pocket: 0.01p + 0.25q. Notice that each expression describes a different characteristic of the change in your pocket. Evaluate each expression for the situation where you have 6 quarters and 7 pennies in your pocket. Type the correct answer in each box. Use numerals instead of words. For the amount of money, do not enter a dollar symbol.

Answer 1

`\Large \boxed {13} \\ \boxed{ 1.57}`

Explanation:

Pennies ⇒
`p`

Quarters ⇒
`q`

First expression describes the total number of coins in the pocket ⇒
`p+q`

Second expression describes the amount of money in dollars in the pocket ⇒
`0.01p+0.25q`

There are 6 quarters and 7 pennies in the pocket.

First expression :

`p+q \\ 7 + 6 = 13`

Second expression :

`0.01p+0.25q \\ 0.01(7)+0.25(6) \\ 0.07+1.5 =1.57`

Answer 2

13

1.57

Explanation:

So we have two equations regarding the number of quarters q and the number of pennies p:

`p+q`

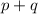
Which represents the total amount of coins and

`0.01p+0.25q`

Which represents the total amount of money in dollars.

So we are asked to evaluation each expression for the situation in which we have 6 quarters and 7 pennies. Thus, plug 6 in for q and 7 in for p:

`p+q\\(7)+(6)=13`

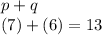
This tells us that we have 16 coins in total.

`0.01(7)+0.25(6)\\=0.07+1.50\\=1.57`

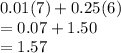
This tells us that we have a total amount of $1.57.