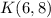Question

The midpoint of JK is M(8,9). One endpoint is J(10, 10). Find the coordinates of the other

endpoint K.
Write the coordinates as decimals or integers.
K= (1,7)

Answer 1

`K(6,8)`

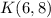
Explanation:

Given

Midpoint, M = (8,9)

J = (10,10)

Required

Find K

The midpoint of segments is calculated using;

`M(x,y) = ((x_1 + x_2)/(2), (y_1 + y_2)/(2))`

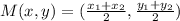
Where
`x = 8` and
`y = 9`

Take J as J(x1,y1);

So:

`x_1 = 10`
`y_1 = 10`

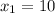
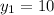
Substitute values for x and y in the given formula

`(8,9) = ((10 + x_2)/(2), (10 + y_2)/(2))`

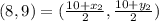
Solving for x2, we have

`8 = (10 + x_2)/(2)`

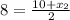
Multiply both sides by 2

`2 * 8 = (10 + x_2)/(2) * 2`

`2 * 8 = 10 + x_2`

`16 = 10 + x_2`

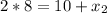
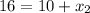
Make x2 the subject of formula

`x_2 = 16 - 10`

`x_2 = 6`

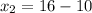
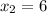
Solving for y2

`9 = (10 + y_2)/(2)`

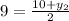
Multiply both sides by 2

`2 * 9 = (10 + y_2)/(2) * 2`

`2 * 9 = 10 + y_2`

`18 = 10 + y_2`

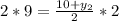
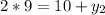
Make y2 the subject of formula

`y_2 = 18 - 10`

`y_2 = 8`

So,

`(x_2,y_2) = (6,8)`

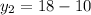
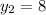
So, the coordinates of K is

`K(6,8)`