Question

Find the quotient of n(x) = 6x^5y^7 and m(x) = 2x^3y

a. Is n(x) a polynomial? If not, give an explanation.
b. Is m(x) a polynomial? If not, give an explanation.
c. Is the quotient a polynomial? If not, give an explanation.
d. If the quotient is a polynomial, identify type and degree.


Pls help with this!!!

Answer 1

a. Yes

b. Yes

c. Yes

d. Degree 8

Explanation:

a. Yes, n(x) is a polynomial of one single term (also called monomial) because it contains variables raised to positive integers.

b. Yes, m(x) is a polynomial of also one single term (also called monomial) because it contains variables raised to positive integers.

c. The quotient of n(x) / m(x) can be reduced to a polynomial of one single term as follows:

`(n(x))/(m(x)) =(6\,x^5\,y^7)/(2\,x^3\,y) =3\,x^2\,y^6`

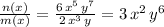
which as can be seen, also contains variables raised to positive integers.

d. The degree of the polynomial resultant is the addition of the powers of all variables present (x and y) which results in: 2 + 6 = 8

Therefore the degree of this polynomial is 8.