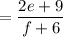Step-by-step explanation
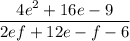
⇒ First, factor the numerator by grouping:
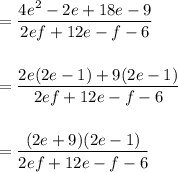
⇒ Now, factor the denominator by grouping:

⇒ We must determine which values of e and f are unacceptable, meaning, will make this expression undefined. These will be the values of e and f that make the denominator equal to 0.
- ⇒ To find these values, let's set each term in the denominator equal to 0, and solve for e and f.
 ⇒
⇒
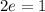 ⇒
⇒
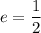
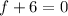 ⇒
⇒
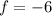
- ⇒ The restrictions for e and f include
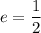 and
and
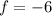 .
.
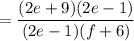
⇒ Reduce values in the numerator and denominator:

Answer