Answer:
If the frequency of the source is increased the current in the circuit will decrease.
Step-by-step explanation:
The current through the circuit is given as;
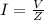
Where;
V is the voltage in the AC circuit
Z is the impedance
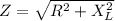
Where;
R is the resistance
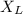 is the inductive reactance
is the inductive reactance
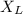 = ωL = 2πfL
= ωL = 2πfL
where;
L is the inductance
f is the frequency of the source
Finally, the current in the circuit is given as;

From the equation above, an increase in frequency (f) will cause a decrease in current (I).
Therefore, If the frequency of the source is increased the current in the circuit will decrease.