Question

Seven apple women, possessing respectively 20, 40, 60, 80, 100, 120, and 140

apples, went to market and sold all their apples at the same price, and each
received the same sum of money. What was the price?

Answer 1

The amount each took home was 20 unit currency.

Explanation:

We are given that Seven apple women, possessing respectively 20, 40, 60, 80, 100, 120, and 140 apples, went to market and sold all their apples at the same price, and each received the same sum of money.

Formula :
`a * n + (n - 1)`,
`(a + b)\cdot n + (n - 2)`,
`(a + 2 \cdot b) \cdot n + (n - 3)`,
`(a + 3 \cdot b) \cdot n + (n - 4)`,
`(a + 4 \cdot b) \cdot n + (n - 5)`,
`(a + 5 \cdot b) \cdot n + (n - 6)`,
`(a + 6 \cdot b) \cdot n + (n - 7)`

`a \cdot n + (n - 1) = 20`

`(a + b) \cdot n + (n - 2)=40`

`(a + 2 \cdot b) \cdot n + (n - 3) = 60`

`(a + 3 \cdot b) \cdot n + (n - 4) = 80`

`(a + 4 \cdot b) \cdot n + (n - 5) = 100`

`(a + 5 \cdot b) \cdot n + (n - 6) = 120`

`(a + 6 \cdot b) \cdot n + (n - 7) = 140`

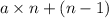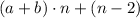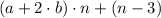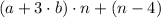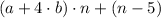On solving :

n = 7, a = 2, b = 3

So,

`2 * 7 + 6 = 20`

`5 * 7 + 5=40`

`8 * 7 + 4 = 60`

`11 * 7 + 3 = 80`

`14 * 7 + 2 = 100`

`17 * 7 + 1 = 120`

Based on market pricing if groups of apples are sold at 1 unit currency for 7, and extras are sold for 3 unit currency per extra 1, we have the amount :

2×1 + 6×3 = 20

5×1 + 5×3=20

8×1 + 4×3 = 20

11×1 + 3×3 = 20

14×1 + 2×3 = 20

17×1 + 1×3 = 20

20×1 = 20

Therefore, the amount each took home was 20 unit currency.