
The AP would be like:
- 1, 3, 5, 7........[50 terms]
Now,
➝ First term = 1
➝ Common difference = 2
➝ No. of terms = 50
By using formula,
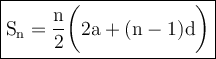
Here,
- a = First term
- n = number of terms
- d = common difference
- Sn = sum of n terms
Proceeding further,
➝ S50 = 50/2{ 2 × 1 + (50 - 1)2 }
➝ S50 = 25{ 2 + 49 × 2 }
➝ S50 = 25{ 100 }
➝ S50 = 2500
⛈️ Sum of 50 terms of AP = 2500
Shortcut trick:- n^2
Then, Sum of 50(n) terms = 50^2 = 2500
☘️ Hence, solved !!
━━━━━━━━━━━━━━━━━━━━