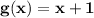Question

Write a function g whose graph represents a reflection in the y-axis of the graph F(x)=-x+1

Answer 1

`\bold{g(x) = x+1}`

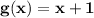
Explanation:

Given a function:

`F(x) =-x+1`

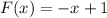
To find:

Function
`g` whose graph is a reflection in the y -axis for the given function = ?

Solution:

First of all, let us learn about the reflection of a point (
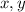`x, y`) in y axis.

For example, let us consider a point (3, 2) on the coordinate axis.

The reflection of this point in y axis will be (-3, 2)

OR

In other words, To find the image of a point in y axis:

the x coordinate is made of negative sign and y coordinate remains the same.

A line is nothing but a number of points joined together.

So, if we want to find reflection of a line or linear function in y axis, we just make the sign of x as negative.

Given that
`F(x) =-x+1`

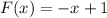
We can write it as:

`F(x)=y =-x+1`

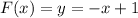
To find its reflection:

`g(x) = - (-x)+1\\\Rightarrow \bold{g(x) = x+1}`

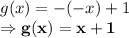
Please refer to the attached graphs of
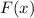`F(x)` and
`g(x)` .

So, the answer is:

`\bold{g(x) = x+1}`