Question

What is the maximum wavelength of incident light that can produce photoelectrons from silver? The work function for silver is Φ=2.93 eV.

Answer 1

The maximum wavelength of incident light that can produce photoelectrons from silver is 423.5 nm.

Step-by-step explanation:

Given;

work function of silver, Φ = 2.93 eV = 2.93 x 1.602 x 10⁻¹⁹ J = 4.6939 x 10⁻¹⁹ J

Apply Einstein Photo electric effect;

E = K.E + Ф

Where;

E is the energy of the incident light

K.E is the kinetic of electron

Ф is the work function of silver surface

For the incident light to have maximum wavelength, the kinetic energy of the electron will be zero.

E = Ф

hf = Ф

`h(c)/(\lambda) = \phi`

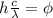
where;

c is speed of light = 3 x 10⁸ m/s

h is Planck's constant, = 6.626 x 10⁻³⁴ J/s

λ is the wavelength of the incident light

`\lambda = (hc)/(\phi)\\\\\lambda =(6.626*10^(-34) *3*10^8)/(4.6939*10^(-19)) \\\\\lambda = 4.235 *10^(-7) \ m\\\\\lambda = 423.5 *10^(-9) \ m\\\\\lambda = 423.5 \ nm`

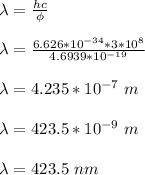
Therefore, the maximum wavelength of incident light that can produce photoelectrons from silver is 423.5 nm.