Explanation:
Hey,there!!!
Let's simply work with it,
We have,
One point is (x2,y2) and midpoint is(x,y).
Now, let's use midpoint formulae,

Now, let's equate with their corresponding elements we get,
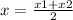
now, 2x=x1+x2
or, x1=2x-x2
and
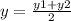
now,
2y = y1+y2
or, y1 = 2y-y2
Therefore, the answer is (x1,y1)= { (2x-x2),(2y-y2) }
Hope it helps...