Answer:
n = 24
Explanation:
Given the fraction:
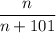
To find:
Smallest positive integer
 such that the fraction is equal to a terminating decimal.
such that the fraction is equal to a terminating decimal.
Solution:
The rule that a fraction is equal to a terminating decimal states that, the denominator must contain factors of only 2 and 5.
i.e. Denominator must look like
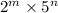 , only then the fraction will be equal to a terminating decimal.
, only then the fraction will be equal to a terminating decimal.
Now, let us have a look at the denominator,
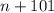
Let us use hit and trial method to find the value of
 as positive integer.
as positive integer.
n = 1, denominator becomes 102 =
 not of the form
not of the form
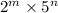 .
.
n = 4, denominator becomes 105 =
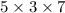 not of the form
not of the form
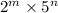 .
.
n = 9, denominator becomes 110 =
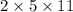 not of the form
not of the form
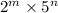 .
.
n = 14, denominator becomes 115 =
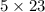 not of the form
not of the form
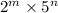 .
.
n = 19, denominator becomes 120 =
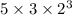 not of the form
not of the form
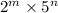 .
.
n = 24, denominator becomes 125 =
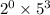 It is of the form
It is of the form
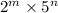 .
.
So, the answer is n = 24