Answer:
Weight 0.4326 g of sodium bicarbonate and 0.5141 g of sodium carbonate, dissolve it in distilled water and then bring the solution to a final volume of 50.0 mL using distilled water.
Step-by-step explanation:
The pH of a buffered solution can be calculated using the Henderson-Hasselbalch equation:
![pH = pKa + log(([Na_(2)CO_(3)])/([NaHCO_(3)]))](https://img.qammunity.org/2021/formulas/chemistry/college/5rgctjq8bw34ertfpotat1m010g6fs9sbc.png)
We have that pH = 10.3 and the Ka is 4.7x10⁻¹¹, so:
![10.3 = -log(4.7 \cdot 10^(-11)) + log(([Na_(2)CO_(3)])/([NaHCO_(3)]))](https://img.qammunity.org/2021/formulas/chemistry/college/2nhi4t9e659gzp0ivxyyv9et17rdet6bng.png)
![([Na_(2)CO_(3)])/([NaHCO_(3)]) = 0.94](https://img.qammunity.org/2021/formulas/chemistry/college/cld0xksrb427jmqcmhzz7r9mdq28llmc4v.png) (1)
(1)
Also, we know that:
![[Na_(2)CO_(3)] + [NaHCO_(3)] = 0.20 M](https://img.qammunity.org/2021/formulas/chemistry/college/zsuxek5b3jv15vbo3dwhpja9p2vrmwlv0i.png) (2)
(2)
From equation (2) we have:
![[Na_(2)CO_(3)] = 0.20 - [NaHCO_(3)]](https://img.qammunity.org/2021/formulas/chemistry/college/666jonjqva4vqy962elh22dojzippfh03z.png) (3)
(3)
By entering (3) into (1):
![(0.20 - [NaHCO_(3)])/([NaHCO_(3)]) = 0.94](https://img.qammunity.org/2021/formulas/chemistry/college/t9ligzkptoh6hts7vhsbjpg4beguwned2p.png)
![0.94*[NaHCO_(3)] + [NaHCO_(3)] = 0.20](https://img.qammunity.org/2021/formulas/chemistry/college/199s86pqdhrkai74a231wlbnvrp3wfyjsz.png)
![[NaHCO_(3)] = 0.103 M](https://img.qammunity.org/2021/formulas/chemistry/college/qwh9cc9j2fdcuft5bpekz3303l7bjt8vbb.png)
Hence, the [Na_{2}CO_{3}] is:
![[Na_(2)CO_(3)] = 0.20 - [NaHCO_(3)] = 0.20 M - 0.103 M = 0.097 M](https://img.qammunity.org/2021/formulas/chemistry/college/b4k11d76tox49c38tswbbs38leg77vqc1k.png)
Now, having the concentrations and knowing the volume of the buffer solution we can find the mass of the sodium carbonate and the sodium bicarbonate, as follows:
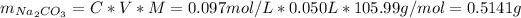
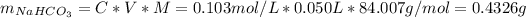
Therefore, to prepare 50.0 mL of a 0.20 M solution that is buffered to a pH of 10.3 we need to weight 0.4326 g of sodium bicarbonate and 0.5141 g of sodium carbonate, dissolve it in distilled water and then bring the solution to a final volume of 50.0 mL using distilled water.
I hope it helps you!