Question

A sample of argon gas (molar mass 40 g) is at four times the absolute temperature of a sample of hydrogen gas (molar mass 2 g). Find the ratio of the rms speed of the argon molecules to that of the hydrogen. Assume hydrogen molecule has only translational degree of freedom.

Answer 1

Ratio of Vrms of argon to Vrms of hydrogen = 0.316 : 1

Step-by-step explanation:

The root-mean-square speed measures the average speed of particles in a gas, and is given by the following formula:

Vrms =
`√(3RT/M)`

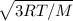
where R is molar gas constant = 8.3145 J/K.mol, T is temperature in kelvin, M is molar mass of gas in Kg/mol

For argon, M = 40/1000 Kg/mol = 0.04 Kg/mol, T = 4T , R = R

Vrms = √(3 * R *4T)/0.04 = √300RT

For hydrogen; M = 1/1000 Kg/mol = 0.001 Kg/mol, T = T, R = R

Vrms = √(3 * R *T)/0.001 = √3000RT

Ratio of Vrms of argon to that of hydrogen = √300RT / √3000RT = 0.316

Ratio of Vrms of argon to that of hydrogen = 0.316 : 1