Answer:
the middle 50% of most lengths of pregnancies ranges between 255.62 days and 274.38 days
Explanation:
Given that :
Mean = 265
standard deviation = 14
The formula for calculating the z score is
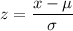
x = μ + σz
At middle of 50% i.e 0.50
The critical value for
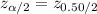
From standard normal table
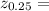 + 0.67 or -0.67
+ 0.67 or -0.67
So; when z = -0.67
x = μ + σz
x = 265 + 14(-0.67)
x = 265 -9.38
x = 255.62
when z = +0.67
x = μ + σz
x = 265 + 14 (0.67)
x = 265 + 9.38
x = 274.38
the middle 50% of most lengths of pregnancies ranges between 255.62 days and 274.38 days