Question

A political candidate has asked his/her assistant to conduct a poll to determine the percentage of people in the community that supports him/her. If the candidate wants a 10% margin of error at a 95% confidence level, what size of sample is needed

Answer 1

The desired sample size is 97.

Explanation:

Assume that 50% people in the community that supports the political candidate.

It is provided that the candidate wants a 10% margin of error (MOE) at a 95% confidence level.

The confidence interval for the population proportion is:

`CI=\hat p\pm z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

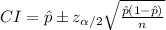
Then the margin of error is:

`MOE= z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

Compute the critical value of z as follows:

`z_(\alpha/2)=z_(0.05/2)=z_(0.025)=1.96`

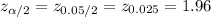
*Use a z-table.

Compute the sample size as follows:

`MOE= z_(\alpha/2)\sqrt{(\hat p(1-\hat p))/(n)}`

`n=[(z_(\alpha/2)* √(\hat p(1-\hat p)) )/(MOE)]^(2)`

`=[(1.96* √(0.50(1-0.50)) )/(0.10)^(2)\\\\=[9.8]^(2)\\\\=96.04\\\\\approx 97`

Thus, the desired sample size is 97.