Answer:

Explanation:
Area of Octagon A = 4 m²
Side length of Octagon A = a
Area of Octagon B = 9 m²
Side length of Octagon B = b
The scale factor of their side lengths =
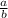
According to the area of similar polygons theorem,

Thus,

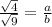
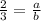
Scale factor of their sides =
