Question

A thick wire with a radius of 4.0 mm carries a uniform electric current of 1.0 A, distributed uniformly over its cross-section. At what distance from the axis of the wire, and greater than the radius of the wire, is the magnetic field strength equal to that at a distance 2.0 mm from the axis. distance

Answer 1

8 mm

Step-by-step explanation:

From the information given:

The Ampere circuital law can be used to estimate the magnetic field strength at two points when the distance is less than the radius and when the distance is greater than the radius.

when the distance is less than the radius ; we have:

`B_1 = ( \mu_o * I * r)/(2 * \pi * \ d^2)`

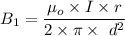
when the distance is greater than the radius; we have:

`B_2 = (\mu_o \ * I)/(2 \ * \pi * R)`

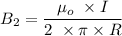
Equating both equations together ; we have :

`( \mu_o * I * r)/(2 * \pi * \ d^2) = (\mu_o \ * I)/(2 \ * \pi * R)`

`(1)/(R)= (r)/(d^2)`

`R= (d^2)/(r)`

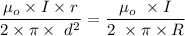
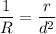
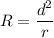
where; d = radius of the wire and r = distance;

`R =(4^2)/(2)`

`R =(16)/(2)`

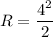
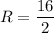
R = 8 mm