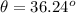Answer:
The angle is
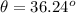
Step-by-step explanation:
From the question we are told that
The mass is
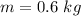
The radius is
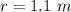
The speed is
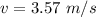
According to the law of energy conservation
The potential energy of the mass at the top is equal to the kinetic energy at the bottom i.e
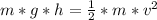
=>
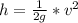
Here h is the vertical distance traveled by the mass which is also mathematically represented as
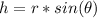
So
![\theta = sin ^(-1) [ (1)/(2* g* r ) * v^2]](https://img.qammunity.org/2021/formulas/physics/college/kogkkdl7cjdt39ortgr5y5ov73z015wr9l.png)
substituting values
![\theta = sin ^(-1) [ (1)/(2* 9.8* 1.1 ) * (3.57)^2]](https://img.qammunity.org/2021/formulas/physics/college/1xx0qondr6sfhhus1gly457ufefnrd8e82.png)