Question

A narrow beam of light containing red (660 nm) and blue (470 nm) wavelengths travels from air through a 1.00 cm thick flat piece of crown glass and back to air again. The beam strikes at an incident angle of 30 degrees. (a) At what angles do the two colors emerge

Answer 1

The color blue emerges at 19.16° and the color red emerges at 19.32°.

Step-by-step explanation:

The angle at which the two colors emerge can be calculated using the Snell's Law:

`n_(1)sin(\theta_(1)) = n_(2)sin(\theta_(2))`

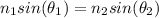
Where:

n₁ is the refractive index of the incident medium (air) = 1.0003

n₂ is the refractive index of the refractive medium:

blue light in crown glass = 1.524

red light in crown glass = 1.512

θ₁ is the angle of the incident light = 30°

θ₂ is the angle of the refracted light

For the red wavelengths we have:

`\theta_(2) = arcsin((n_(1)sin(\theta_(1)))/(n_(2))) = arcsin((1.0003*sin(30))/(1.512)) = 19.32 ^(\circ)`

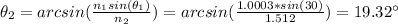
For the blue wavelengths we have:

`\theta_(2) = arcsin((n_(1)sin(\theta_(1)))/(n_(2))) = arcsin((1.0003*sin(30))/(1.524)) = 19.16 ^(\circ)`

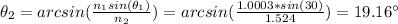
Therefore, the color blue emerges at 19.16° and the color red emerges at 19.32°.

I hope it helps you!