Question

A police car is located 40 feet on a small straight road perpendicular to main highway. A red car is driving along a highway in the direction of an intersection with that small road and is 180 feet away from the intersection. The police radar reads that the distance between the police car and the red car (this distance is straight between them - not on either road) is decreasing at a rate of 100 feet per second. How fast is the red car actually traveling along the road

Answer 1

the red car is traveling at 102.44 ft/s along the road

Explanation:

From the given information:

Let consider p be how far the car is up the road and q be how far the police is off the road.

Also, suppose that r is the distance between the police and the car.

Then, we can have a right triangle in which we can use the Pythagorean Theorem to calculate the r (distance between the cop and the car)

NOW,

p² + q² = r²

r² = 40² + 180²

r² = 1600 + 32400

r² = 34000

r =
`√(34000)`

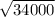
r = 184.39

If we consider q' to be how fast the car is traveling down the road.

And, p' be how fast the police is traveling toward the road.

r' be how fast the distance between the police and the car is changing.

then , the derivative of our equation, p² + q² = r² with respect to time can now be:

2p(p') +2q(q') = 2r(r')

p(p') +q(q') = r(r')

By replacing our values; we have:

40(0) +180(y') =
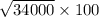`√(34000) * 100` (given that the police is not moving p' =0)

180(y') =
`√(34000) * 100`

`(y') = (√(34000) * 100)/(180)`

`(y') = (184.39 * 100)/(180)`

`\mathbf{(y') = 102.44 \ ft/s}`

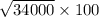
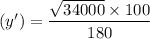
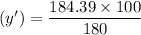
the red car is traveling at 102.44 ft/s along the road