Question

An IQ test is designed so that the mean is 100 and the standard deviation is for the population of normal adults. Find the sample size necessary to estimate the mean IQ score of statistics students such that it can be said with ​% confidence that the sample mean is within IQ points of the true mean. Assume that and determine the required sample size.

Answer 1

Complete Question

An IQ test is designed so that the mean is 100 and the standard deviation is 24 for the population of normal adults. Find the sample size necessary to estimate the mean IQ score of statistics students such that it can be said with 95% confidence that the simple mean is with in 3 IQ points of the true mean. Assume that standard deviation = 24 and determine the required sample size using technology. Determine if this is a reasonable sample size for a real world calculation.

The required sample size ______ (round up to the nearest integer.

Answer:

The sample size is
`n = 246`

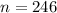
Explanation:

From the question we are told that

The mean is
`\mu = 100`

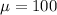
The standard deviation is
`\sigma = 24`

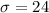
The margin of error is
`E = 3`

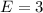
Given that the confidence level is 95% then the level of significance is mathematically evaluated as

`\alpha = 100 - 95`

`\alpha = 5\%`

=>
`\alpha = 0.05`

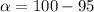
Next we obtain the critical value of
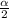`( \alpha )/(2)` from the normal distribution table the value is

`Z_{(\alpha )/(2) } = 1.96`

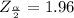
The sample size is evaluated as

`n = [ \frac{ Z_{(\alpha )/(2) } * \sigma }{E }]^2`

=>
`n = [ ( 1.96 * 24 )/(3 )]^2`

=>
`n = 246`

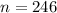
to check if this n is applicable in real world then we calculate E and compare it with the given E