Question

A mass M is attached to an ideal massless spring. When this system is set in motion with amplitude A, it has a period T. What is the period if the amplitude of the motion is doubled

Answer 1

The period of the motion will still be equal to T.

Step-by-step explanation:

for a system with mass = M

attached to a massless spring.

If the system is set in motion with an amplitude (distance from equilibrium position) A

and has period T

The equation for the period T is given as

`T = 2\pi \sqrt{(M)/(k) }`

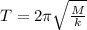
where k is the spring constant

If the amplitude is doubled, the distance from equilibrium position to the displacement is doubled.

Increasing the amplitude also increases the restoring force. An increase in the restoring force means the mass is now accelerated to cover more distance in the same period, so the restoring force cancels the effect of the increase in amplitude. Hence, increasing the amplitude has no effect on the period of the mass and spring system.