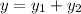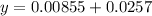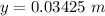Answer:
The distance is
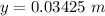
Step-by-step explanation:
From the question we are told that
The distance of separation is

The wavelength is

The distance of the screen is
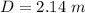
Generally the distance of a fringe from the central maxima is mathematically represented as
![y = [m + (1)/(2) ] * (\lambda * D)/(d)](https://img.qammunity.org/2021/formulas/physics/college/grybd6zyctbwqck6egz8w7ttzuuj19bz0e.png)
For the first dark fringe m = 0
![y_1 = [0 + (1)/(2) ] * (482*10^(-9) * 2.14)/( 60.3*10^(-6))](https://img.qammunity.org/2021/formulas/physics/college/s1kh8in1mdl3h97v27c17o9ks0olvtvgwf.png)

For the second dark fringe m = 1
![y_2 = [1 + (1)/(2) ] * (482*10^(-9) * 2.14)/( 60.3*10^(-6))](https://img.qammunity.org/2021/formulas/physics/college/jmixn8f052aho4h5fa148rrhrr8eqi5246.png)
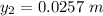
So the distance from the first dark fringe on one side of the central maximum to the second dark fringe on the other side is