Question

If the ac peak voltage across a 100-ohm resistor is 120 V, then the average power dissipated by the resistor is ________

Answer 1

The average power dissipated is 72 W.

Step-by-step explanation:

Given;

peak voltage of the AC circuit, V₀ = 120 V

resistance of the resistor, R = 100 -ohm

The average power dissipated by the resistor is given by;

`P_(avg) = (1)/(2) I_oV_o= I_(rms)V_(rms) = (V_(rms)^2)/(R)`

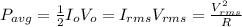
where;

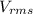`V_(rms)` is the root-mean-square-voltage

`V_(rms) = (V_o)/(√(2)) \\\\V_(rms) = (120)/(√(2))\\\\V_(rms) = 84.853 \ V`

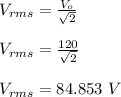
The average power dissipated by the resistor is calculated as;

`P_(avg) = (V_(rms)^2)/(R)\\\\P_(avg) = (84.853^2)/(100)\\\\P_(avg) = 72 \ W`

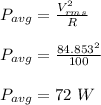
Therefore, the average power dissipated is 72 W.