Question

A company is considering expanding their production capabilities with a new machine that costs $38,000 and has a projected lifespan of 8 years . They estimate the increased production will provide a constant $5,000 per year of additional income . Money can earn 1.7% per year, compounded continuously . Should the company buy the machine

Answer 1

the company should not buy the machine.

Step-by-step explanation:

Given that:

cost of the new machine = $38000

lifespan = 8 years

constant income = 5,000

Interest = 1.7%

no of days = 365

The value of earning at the time of buying can be calculated as follows:

`= (5000)/((1+ (1.7)/(100))^8)+ (5000)/((1+ (1.7)/(100))^7)+(5000)/((1+ (1.7)/(100))^6)+...+ (5000)/((1+ (1.7)/(100))^0)`

`= 5000 \begin {pmatrix} (1)/((1.017)^8)+ (1)/((1.017)^8)+(1)/((1.017)^6)+...+ 1} \end {pmatrix}`

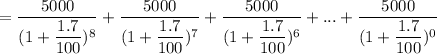
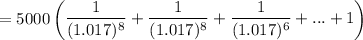
Sum of a Geometric progression
`S=a ((r^n -1))/((r-1))`

`S=((1)/(1.017))^8 (((1.017)^9 -1))/((1.017-1))`

`S= (((1.017)^9 -1))/( (1.017)^8(0.017))`

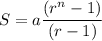
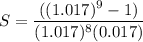
S = 8.4211

The value of earning at the time of buying = (5000 × 8.4211)-$5000

The value of earning at the time of buying = $42105.5 -$5000

The value of earning at the time of buying = $37105.5

The Machine price = $38000

If the value - Machine price > 0, then the company should buy the machine

∴

= $ 37105.5 - $38000

= -$ 894.5

Since the value is negative which is less than zero, then the company should not buy the machine.