Question

Find the doubling time of an investment earning 8% interest if interest is compounded continuously. The doubling time of an investment earning 8% interest if interest is compounded continuously is ____ years.

Answer 1

symbolically, the answer would be t= ln(2)/(.08)

Explanation:

start by writing out your variables:

rate= .08

*dont forget the investment doubles too, thats where 2P is in the bottom equation

equation should look like:

`2P=Pe^(.08t)`

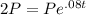
then you solve, so divide P on the right and left:

`(2p)/(p) = (Pe^(.08t))/(p)`

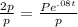
now it looks like:
`2=e^(.08t)`

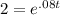
you can take the natural log (ln) of 2 to get the exponent by itself .08t

ln(2)=.08t

then divide .08 to get t by itself

`(ln(2))/(.08) =(.08t)/(.08)`

so symbolically, your equation should be:

`t=(ln(2))/(.08)`

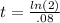
to get t as your answer you can plug this equation into your calculator to get:

t=8.66 years so approximently 8 years

Answer 2

8.66 years

Explanation:

Given that:

Interest rate = 8%

Using the exponential growth function:

A = Ao * e^(rt)

Where A = final amount

Ao = Initial amount

r = growth rate

t = time

Here we are to calculate the time it takes an investment earning 8% interest to double;

rate (r) = 8% = 0.08

2A = A * e^(rt)

Divide both sides by A

2 = e^(rt)

2 = e^(0.08 * t)

2 = e^(0.08t)

In(2) = 0.08t

0.6931471 = 0.08t

Divide both sides by 0.08

0.6931471 / 0.08 = 0.08t / 0.08

8.6643397 = t

t = 8.66 years