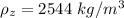Question

A rock has mass 1.80 kg. When the rock is suspended from the lower end of a string and totally immersed in water, the tension in the string is 10.8 N . What is the smallest density of a liquid in which the rock will float?

Answer 1

The density is
`\rho_z = 2544 \ kg /m^3`

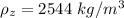
Step-by-step explanation:

From the question we are told that

The mass of the rock is
`m_r = 1.80 \ kg`

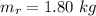
The tension on the string is
`T = 10.8 \ N`

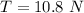
Generally the weight of the rock is

`W = m * g`

=>
`W = 1.80 * 9.8`

=>
`W = 17.64 \ N`

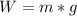
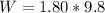
Now the upward force(buoyant force) acting on the rock is mathematically evaluated as

`F_f = W - T`

substituting values

`F_f = 17.64 - 10.8`

`F_f = 6.84 \ N`

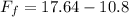
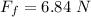
This buoyant force is mathematically represented as

`F_f = \rho * g * V`

Here
`\rho` is the density of water and it value is
`\rho = 1000\ kg/m^3`

So

`V = (F_f)/( \rho * g )`

`V = (6.84)/( 1000 * 9.8 )`

`V = 0.000698 \ m^3`

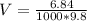
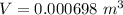
Now for this rock to flow the upward force (buoyant force) must be equal to the length

`F_f = W`

`\rho_z * g * V = W`

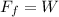
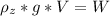
Here z is smallest density of a liquid in which the rock will float

=>
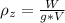
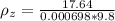
`\rho_z = (W)/( g * V)`

=>
`\rho_z = (17.64)/( 0.000698 * 9.8)`

=>
`\rho_z = 2544 \ kg /m^3`