Question

A study was conducted to compare the effect of three diet types on the milk yield of cows (in lbs). The sample size, sample mean, and sample variance for each method are given below.

Diet A: n1 = 9, X1 = 39.1, s21 = 24.6
Diet B: n2 = 8, X2 = 29.9, s22 = 16.4
Diet C: n3 = 10, X3 = 45.9, s21 = 10.3
(a) Construct an ANOVA table including all relevant sums of squares, mean squares, and degrees of freedom.
(b) Perform an overall F test to determine whether the population means of milk yield are the same or not among the three diet types.

Answer 1

(a) Anova table is attached below.

(b) The population means of milk yield are different among the three diet types

Explanation:

In this case we need to perform a One-way ANOVA to determine whether the effect of three diet types on the milk yield of cows are significantly different or not.

The hypothesis can be defined as follows:

H₀: The effect of three diet types on the milk yield of cows are same.

Hₐ: The effect of three diet types on the milk yield of cows are significantly different.

(a)

The formulas are as follows:

`\text{Grand Mean}=\bar x=(1)/(3)\sum \bar x_(i)\\\\SSB=\sum n_(i)(\bar x_(i)-\bar x)^(2)\\\\SSW=\sum (n_(i)-1)S^(2)_(i)\\\\N=\sum n_(i)\\\\DF_(B)=k-1\\\\DF_(W)=N-k\\\\DF_(T)=N-1\\`

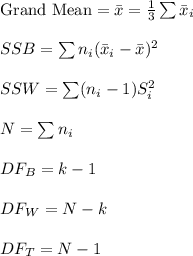
The F critical value is computed using the Excel formula:

F critical value=F.INV.RT(0.05,2,24)

The ANOVA table is attached below.

(b)

The rejection region is defined as follows:

F > F (2, 24) = 3.403

The computed F statistic value is:

F = 34.069

F = 34.269 > F (2, 24) = 3.403

The null hypothesis will be rejected.

Thus, concluding that the population means of milk yield are different among the three diet types