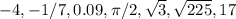Answer:
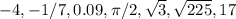
Explanation:
So we have the numbers:

(And without using a calculator) approximate each of the values.
π is around 3.14, so π/2 is around 1.57.
17 squared is 289, so 1.7 squared is 2.89. Thus, the square root of 13 is somewhere between 1.7 and 1.8.
-1/7 can be divided to be about -0.1429...
And the square root of 225 is 15.
Now, use the approximations to place the numbers:

The smallest is -4.
Next is -1/7 or about -0.14
Followed by the first positive, 0.09.
And then with π/2 or 1.57
And then a bit bigger with the square root of 3 or 1.7.
And then with the square root of 225 or 15.
And finally the largest number 17.
Thus, the correct order is: