Answer:
A
The correct option is B
B
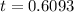
C
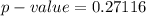
D
The correct option is D
Explanation:
From the question we are told that
The sample size is
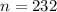
The number that developed nausea is X = 50
The population proportion is p = 0.20
The null hypothesis is
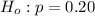
The alternative hypothesis is
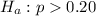
Generally the sample proportion is mathematically represented as
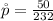
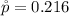
Generally the test statistics is mathematically represented as
=>
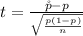
=>
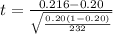
=>
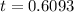
The p-value obtained from the z-table is
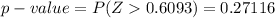
Given that the
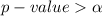 then we fail to reject the null hypothesis
then we fail to reject the null hypothesis