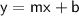Answer:
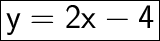
Explanation:
The given equation is:
y = 2x + 3
Where Slope = m = 2 , Y-intercept = b = 3
Parallel lines have equal slopes
So, Slope of new line = m = 2
Now, Finding y-intercept:
Given Point = (x,y) = (1,-2)
So, x = 1 , y = -2
Putting m, x and y in standard form of equation to get b:
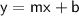

Subtracting 2 to both sides
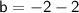
b = -4
So, the standard form og equation for the new line is :