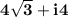Answer:
Explanation:
Given complex number is:
![[2(cos10^\circ + i sin10^\circ)]^3](https://img.qammunity.org/2021/formulas/mathematics/college/uo3icmpyb8htmreqxye8ysbsd0baannbhf.png)
To find:
Answer in rectangular form after using De Moivre's theorem = ?
i.e. the form
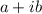 (not in forms of angles)
(not in forms of angles)
Solution:
De Moivre's theorem provides us a way of solving the powers of complex numbers written in polar form.
As per De Moivre's theorem:

So, the given complex number can be written as:
![[2(cos10^\circ + i sin10^\circ)]^3\\\Rightarrow 2^3 * (cos10^\circ + i sin10^\circ)^3](https://img.qammunity.org/2021/formulas/mathematics/college/ozuwo5dv21vuk37c2ygaoz1q2fgrjpg3f4.png)
Now, using De Moivre's theorem:
![\Rightarrow 2^3 * (cos10^\circ + i sin10^\circ)^3\\\Rightarrow 8 * [cos(3 *10)^\circ + i sin(3 *10^\circ)]\\\Rightarrow 8 * (cos30^\circ + i sin30^\circ)\\\Rightarrow 8 * (\frac{\sqrt3}2 + i (1)/(2))\\\Rightarrow \frac{\sqrt3}2* 8 + i (1)/(2)* 8\\\Rightarrow \bold{4\sqrt3 + i4}](https://img.qammunity.org/2021/formulas/mathematics/college/sabdfqxm6gq2c4cjb109jets7ky8vrsa2m.png)
So, the answer in rectangular form is: