Answer:
![\sqrt[3]{4}* (\sqrt[3]{16}-10 )](https://img.qammunity.org/2021/formulas/mathematics/college/4wsddocd8pxyn24osjqa9kq7a2b7rc44fz.png)
Explanation:
Let be
![\sqrt[3]{64}-\sqrt[3]{32} * \sqrt[3]{125}](https://img.qammunity.org/2021/formulas/mathematics/college/8fdwogi19bp2tsq2ecs2bzcpy8a3wm6bid.png) , this expression is simplified as follows:
, this expression is simplified as follows:
1)
![\sqrt[3]{64}-\sqrt[3]{32} * \sqrt[3]{125}](https://img.qammunity.org/2021/formulas/mathematics/college/8fdwogi19bp2tsq2ecs2bzcpy8a3wm6bid.png) Given
Given
2)
![\sqrt[3]{4^(3)}-\sqrt[3]{2^(5)}* \sqrt[3]{5^(3)}](https://img.qammunity.org/2021/formulas/mathematics/college/lcrr57c7n2arc5kvwjii67eprw9xya39jv.png) Definition of power
Definition of power
3)
 Definition of n-th root/
Definition of n-th root/
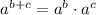 /
/
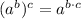
4)
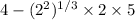
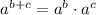 /
/
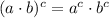
5)
 Multiplication/Definition of power
Multiplication/Definition of power
6)
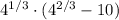 Distributive property/
Distributive property/
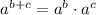
7)
![\sqrt[3]{4}* [(4^(2))^(1/3)-10]](https://img.qammunity.org/2021/formulas/mathematics/college/vqypplly0wnm2m27u8ttsfogltwseabzg7.png)
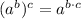 /Definition of n-th root
/Definition of n-th root
8)
![\sqrt[3]{4}* (\sqrt[3]{16}-10 )](https://img.qammunity.org/2021/formulas/mathematics/college/4wsddocd8pxyn24osjqa9kq7a2b7rc44fz.png) Definition of power/Result
Definition of power/Result