Answer:
Third option is the correct answer
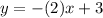
Explanation:
Given functions are:
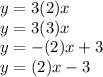
To find:
The function which has a range
?
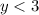Solution:
First of all, let us learn about the terms Domain and Range for a function:
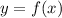
Domain is the value of
which is given as input to the function and gives a valid value of output as
(i.e. function is defined).
Range of a function is the value
that comes as output when given a valid value of
as input.
Now, let us consider the given options above.
Third option is the correct answer.
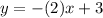
As we can see,
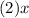is subtracted from 3 so
will have a value which is lesser than 3.
i.e. range will be
.
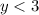No other function has such condition present in it.
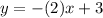is the correct answer.